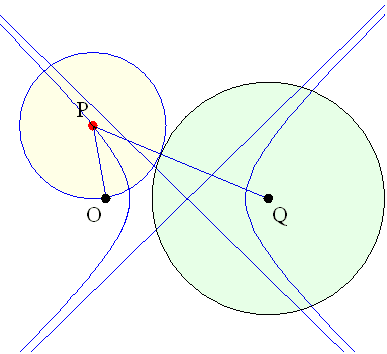
The hyperbola is defined as the set of points for which the difference of distances from two foci (fixed points) is constant.
First, draw a circle whose center is the first focus Q and then draw another circle which is tangent to the fixed circle and passes through the second focus O. Then the center of this second circle belongs to the hyperbola, and traverses the graph.
Note that the eccentricity determines the type of a conic, whether it is an ellipse, parabola, or hyperbola.